Acoustics
displacement is how far the molecule was moved from its initial spot. This concept is what gives us our amplitude (loudness)
- a full 75 player orchestra is capable of generating 70 watts of energy
- there is large range between the instruments when it comes to energy generated. The trombone radiates 100x more sound evergy per second than the horn when both are played fortissimo. The impact of this disparity is reduced by the way the sound hits the ear.
How sound travels
- Sounds are brought to our ears by air. When an object (ex. hitting a snare drumskin) moves, it disturbs the air around it. More specifically, it disrupts the natural motion of air molecules near the object.
- The first upward movement of air (due to the recoil of the drumskin) pushes against the layer of air above it, causing the air to compress between the drumskin and the higher layers of air. After a short time, it is able to expand by pushing the next layers of air outward, thereby compressing the layers above that.
- Meanwhile, the drumskin will fall again, giving the room right above it more volume to fill, so the air expands to fill that space. Consequently, the atmospheric pressure of that air drops (since the same amount of air molecules are now filling a larger space). The air in the layer above experiences lower pressure in the air below it, so it expands downwards. Thus, a pulse of expansion follows the pulse of compression outwards from the drumskin.
- This pattern repeats, and what results is a repeating pulse of compression and expansion we call a soundwave.
- The disturbance of these air molecules creates a domino effect that permeates in all direction outward. This pattern effectively becomes an entity itself, which we call a soundwave.
- Consider at a stadium when fans do The Wave. Each person effectively influences the movement of the person beside them, and so on down the line. In effect, that wave has become its own entity. This is the sound wave.
- If the movement of an object (guitar string) is rapid and regular (ie. consistent), then regular soundwaves will be produced. This is the type of sound that produces tones (a sound with a particular pitch). We associate this type of sound with music.
- note: tone here is used in a physics sense.
A common assumption is that the amplitude of 2 instruments playing together equals the sum of the amplitude of the 2 instruments (ie. 2 guitars is twice as loud as 1 guitar). This is due to the fact that each component of an overall sound may reduce the effective loudness of its neighbors.
Diffraction
Diffraction is the reason why we can still hear sounds if there is a pillar between us and the sound source. The sound wraps around the pillar and soundwaves on both sides join up.
- The pillar creates a sort of sound shadow, whereby some of the higher frequencies are cut out from what we can hear. The further we move away from the pillar, the more out of this shadow we are, and we can hear the sound just as well as if the pillar weren't between us and the sound.
- The width of the pillar and the frequency of the sound impact the degree of diffraction. If the soundwave is longer than the pillar is wide (recall that long soundwaves correspond to lower frequencies), then the sound will have no problem getting to your ears. However, if the pillar is wider than the soundwave, then it will be more difficult to hear.
- this is why when we hear music playing in another room, it sounds like a low-pass filter has been applied to the sound. It's bass heavy because lower frequencies diffract more easily.
Because soundwaves diffract easier as the frequency is dropped, we will notice that when a trombone plays a low note, the intensity of the sound energy radiated forwards does not greatly differ from the intensity of the sound energy radiated backwards
we do not notice much of a difference in loudness whether we are standing in front of them or behind.
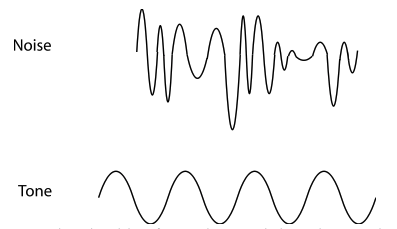
Noise vs Tone
Noise is all frequencies at once
Imagine taking a handful of stones and dropping them into a pond. Each would make its own wave, and those waves would go outward in all directions, crashing into the other waves and distrupting their natural path and canceling them out. This is analogous to noise, which is basically disorganized sound waves (or more simply, disorganized sound).
- This analogy also illustrates how each successive wave from each stone cancels out some of the waves originating from other stones.
Timbre
- def - the characteristic difference in sound between instruments.
- Timbre is created due to the unique combination of different level harmonics.
- ex. a clarinet might have relatively loud 3rd and 8th harmonics. This unique combination is what gives the clarinet its tone.
- unless excessive, vibrato is perceived as an enrichment of timbre, rather than a change in pitch
Why certain notes sound good together
- Say someone plays a note, a middle C. Now someone else plays the note that is twice the frequency of the middle C. Since this second note was already a harmonic of the first note, the sound waves of the two notes reinforce each other and sound good together.
- These are the harmonics found in the A note:
A A E A C# E G A
1 2 3 4 5 6 7 8
- when a sound wave encounters a wall, it does one of 2 things:
- It bounces (reflection) or
- It bends (refraction)
- waves can be trapped by multiple objects facing each other, resulting in standing waves.
- this is how musical instruments achieve pitch (how sharp or flat something is, in a relative sense)
- When a musical tone is played, only a particular set of frequencies is heard. So when you play a note on a piano, what is heard is actually a blend of multiple pitches.
- These pitches are called harmonics. They give the sound its timbre.
Frequency (Hertz— Hz)
- The frequency of a sound is the number of cycles of the vibration which are completed in 1 second.
- A cello playing a low E♭ has a frequency of 78, meaning the string vibrates up and down 78 times per second.
- Musicians use the term pitch.
- all sound waves travel at about the same speed. This means that waves with a shorter wavelength (ie. higher pitch) will arrive at your ear more frequently.
- This aspect of sound (how often a wave gets to your ear) is called frequency.
Harmonic Frequencies
below: the red line represents a sine wave, whereas the superimposed black wave is a guitar. The guitar wave generally follows the sine wave, but there are bumps. The space between the black wave and the red one are the harmonic frequencies that are put out by the guitar.
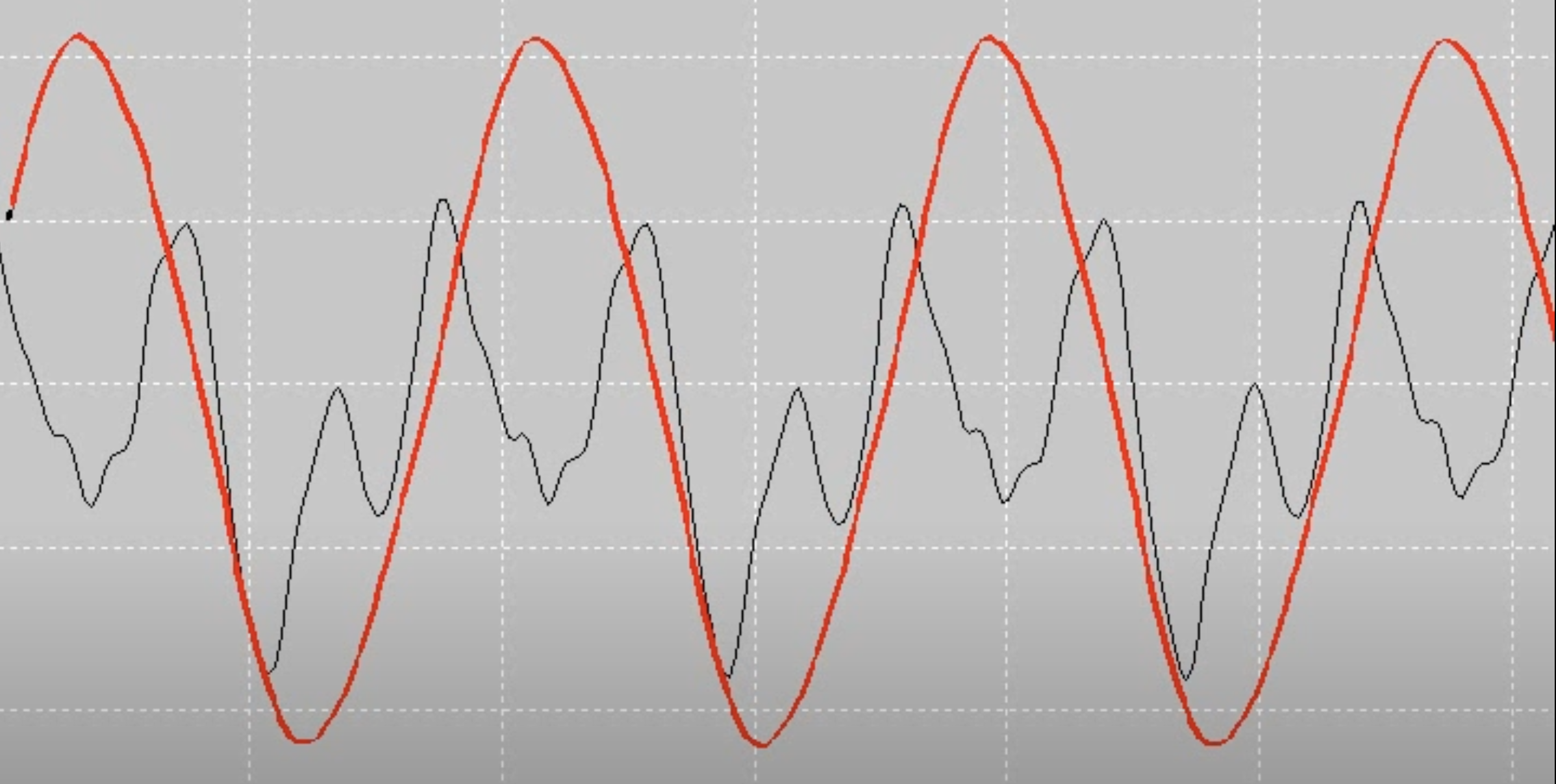
It is the unique harmonic frequencies of each sound that give it its timbre.
below: the soundwave recorded with a microphone at the soundhole will produce a wave that is much more similar to the sine wave.
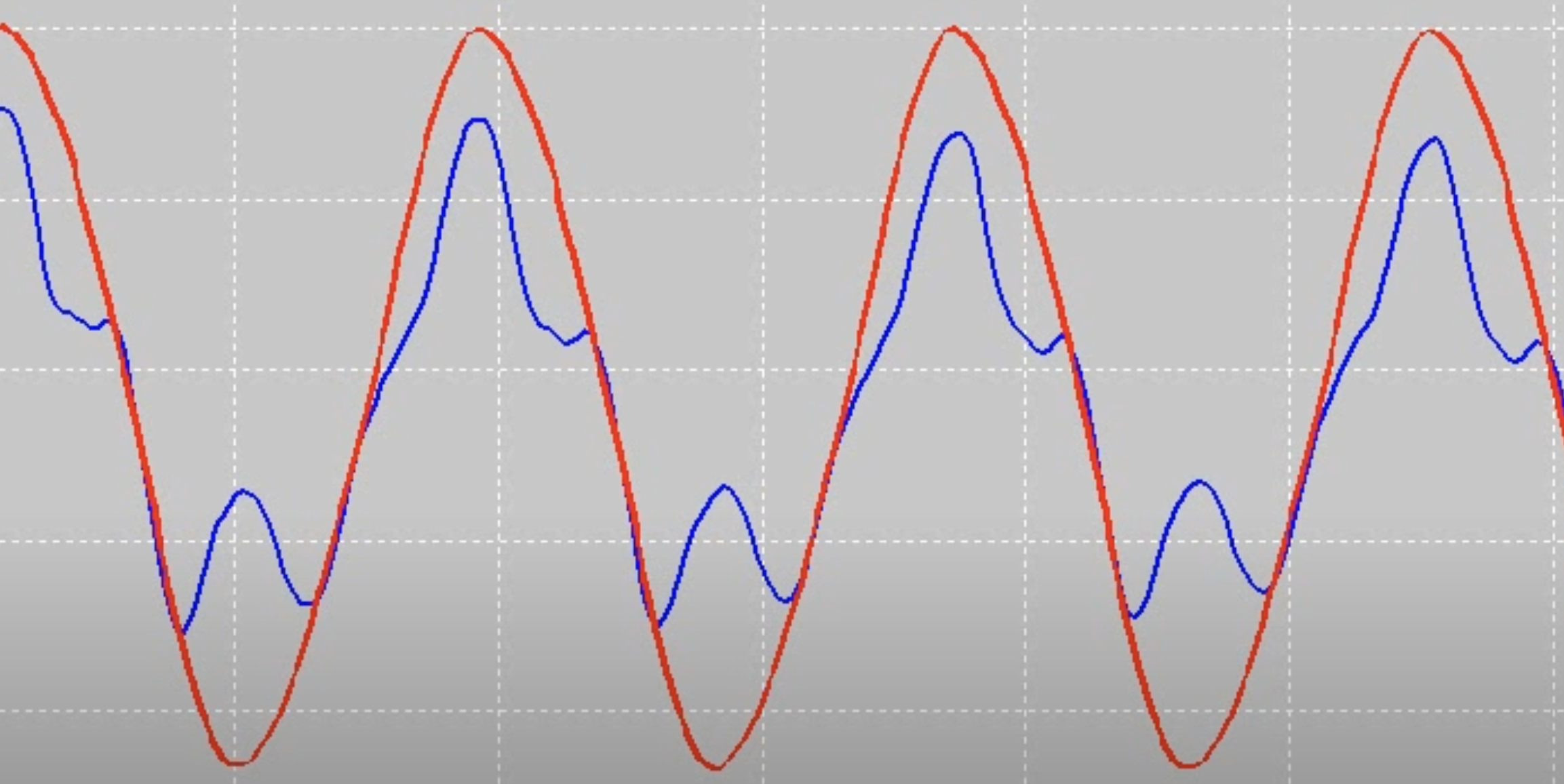
compare this to a microphone at the neck.

This is because the fundamental frequencies are accented over the soundhole, whereas the harmonic frequencies are accented over the neck.
The illustrates how playing an A on guitar and an A on a clarinet will produce the exact same fundamental— it is the harmonics that give each sound its unique timbre.
below: an A on guitar. Notice the fundamental frequency as the leftmost peak, while the others are harmonic frequencies.
- notice that they get closer together as we move higher up the frequency spectrum.
- also notice that past a certain point, harmonics start to become more faint.
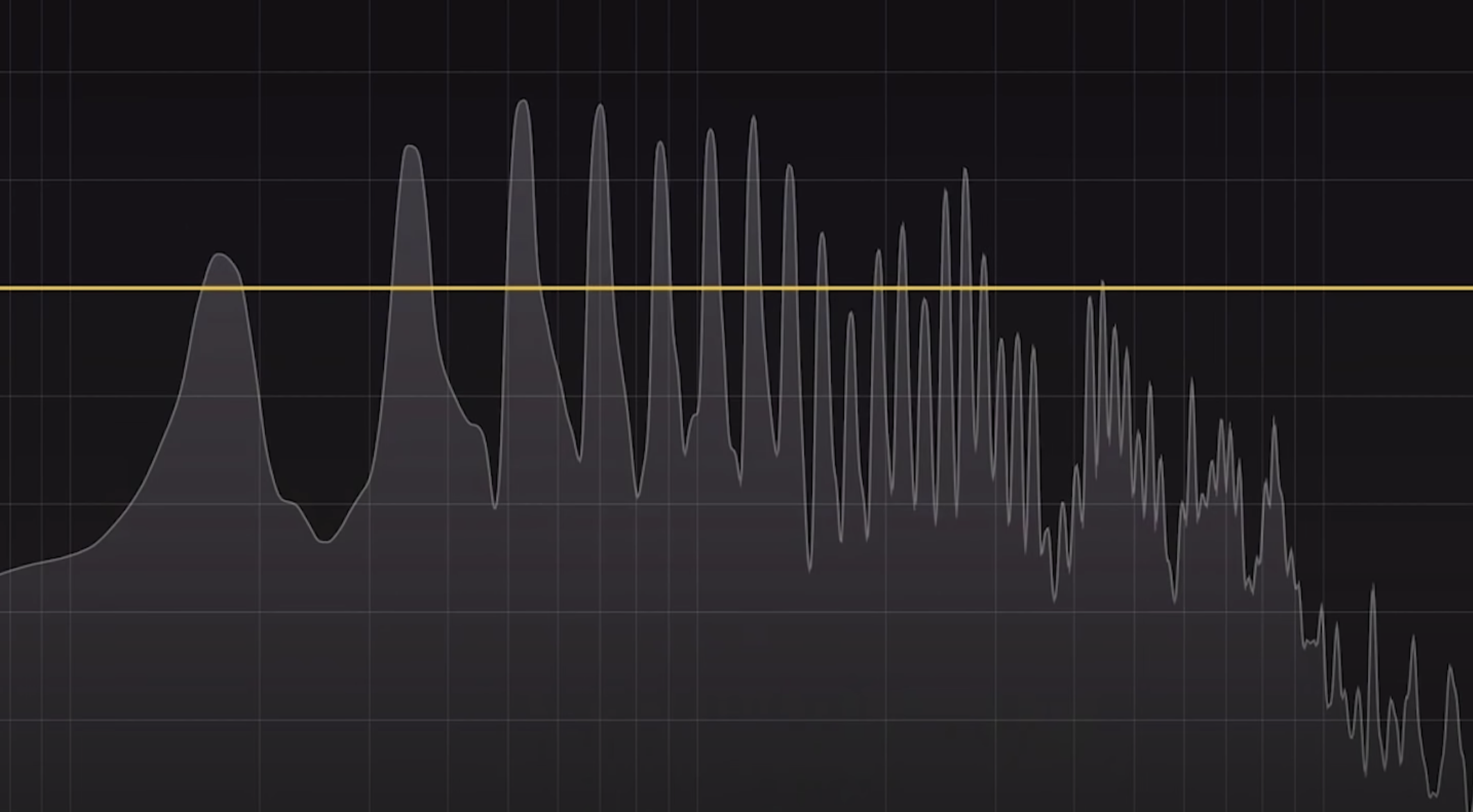
A soundwave that has no harmonic, is a sine wave.
- therefore all harmonics are sine waves.
Every complex sound is made up of 2 or more sine waves added together
- even the slightest change in the timing of a single sine wave will have drastic effects on the resultant complex wave.
The first 6 harmonics (ie. fundmental+5 overtones) make up a major chord.
below: every harmonic note that isn't an octave of the fundamental is slightly out of tune.
- here "out of tune" is in the context of equal temperament, whereby an octave is split into 12 evenly spaced frequency ranges and each represented by a note. Acoustically, the 12 frequency ranges are not equal. Consider that we can describe relationships between tones with ratios (octave is 2:1, 4:3 is a 4th, a tritone is 45:32 etc.). Many of these ratios yield an irrational frequency number. This system of tuning is called just intonation.
- spec: ex. Middle C is at ~260Hz. A perfect 4th (4:3) from C would be at 346.666Hz (260 * 4 / 3)
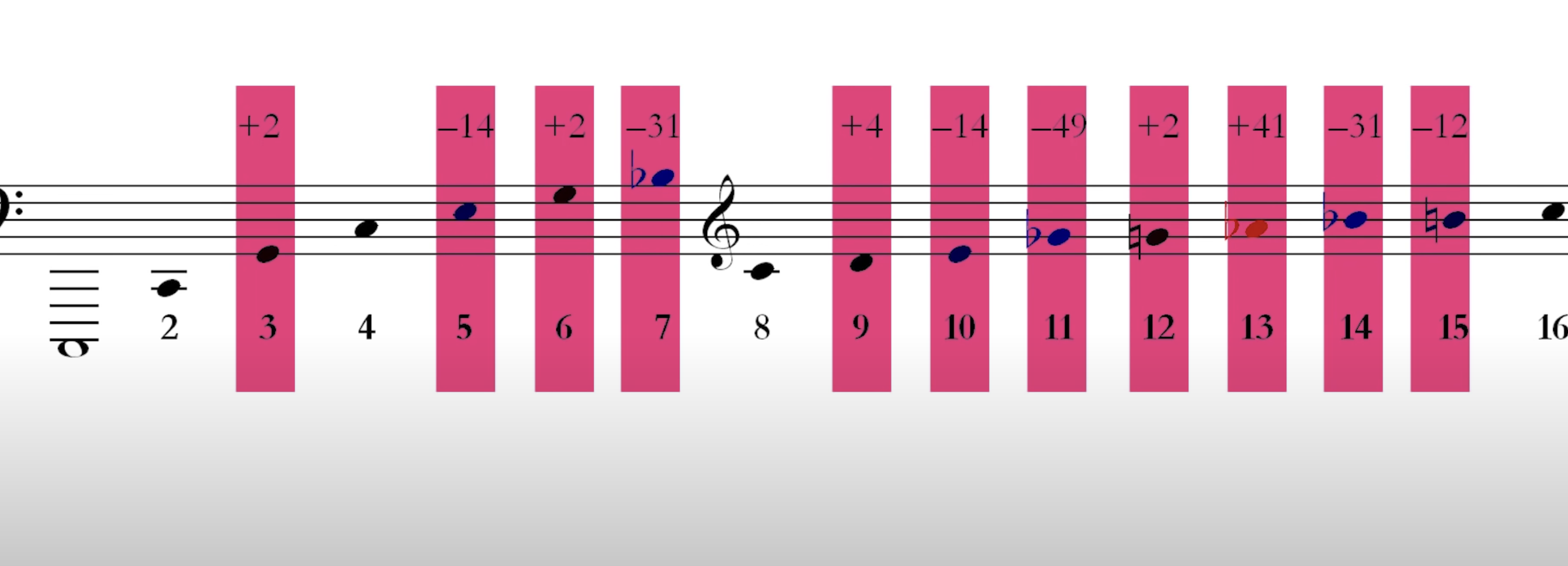
Naturally, if we were using the just intonation method of tuning, our notes would all be perfectly in tune.
If we record the sound of the note, C3, along with the six harmonic components and replay it through an amplifier with treble and bass controls, we can progressively remove the upper harmonics by turning down the treble control. The pitch of the note remains constant, even when all the harmonics except the first have been filtered out, leaving us with a pure tone of pitch C3.
It is tempting to conclude from this experiment that the fundamental gives the pitch, while the upper harmonics merely add tone color. We can disprove this theory By turning down the base control on the amplifier. When we do this, we can progressively remove the lower members of the harmonic series. When the fundamental has been filtered out completely, We still hear the pitch C3, even though the lowest pure tone, reaching our ears now has a pitch of C4.
A set of pure tones fuse into a single pitch, only if they are members of a harmonic serious. If the notes are not harmonically related, each tone is heard separately, and there is no definite feeling of pitch associated with the complete sound.
If we take a C3 and add the following five harmonic frequencies and then remove the C3, The resulting sound will still be perceived as having a pitch of C3.
Not all harmonics contribute equally to establishing the pitch of a sound. For notes in the bass clef, the 4th and 5th harmonics play the most significant role. For notes in the treble clef, the 2nd and 3rd harmonics are of greatest importance. Interestingly, it is only above C7 that wthe 1st harmonic is the dominant factor in establishing the pitch
The closer to a pure sine wave the tone, the more difficult it is to ascertain which octave the note is being played in
- a recorder often sounds an octave lower than it actually is
Equal Temperament
If we are tuning our guitar with a tuner, then our instrument is tuned according to equal temperament. If we are using harmonics to tune, then we are using just intonation.
Notes in equal temperament aren't the most pleasing "versions" of those notes, but they will sound approximately right in any context.
- this is why if we make our open C chord perfectly in tune and then go play an E chord, it sounds out of tune.
- for this reason, it might be advisable to tune to concert pitch with a tuner, then make any fine adjustments needed to make the guitar sound good in whatever key you're playing in.
Inverse Square Law of Sound
The inverse square law states that "with every doubling of distance away from the sound source, the sound will be four times less intense."
- Consider that we can visualize sound as an expanding sphere, since it will extend out in all directions from the source. After a sound has been made, the same amount of energy will exist in that sphere no matter if we are close to the source (small sphere), or if we are further away (larger sphere). Therefore, the larger the sphere is, the more surface area the energy must be spread over, resulting in less energy at any single point.
- Also consider that when we double the radius of a sphere, the surface area quadruples. This means that when we move from 1m to 2m away from a sound source, the strength of the sound wave at any single point on the surface of the sphere is actually 1/4. Since our ears/microphones only pick up sound from a small portion of the entire surface area of the soundwave, this effectively means that the sound pressure level of that soundwave is 4x less as we double our distance from that sound source.
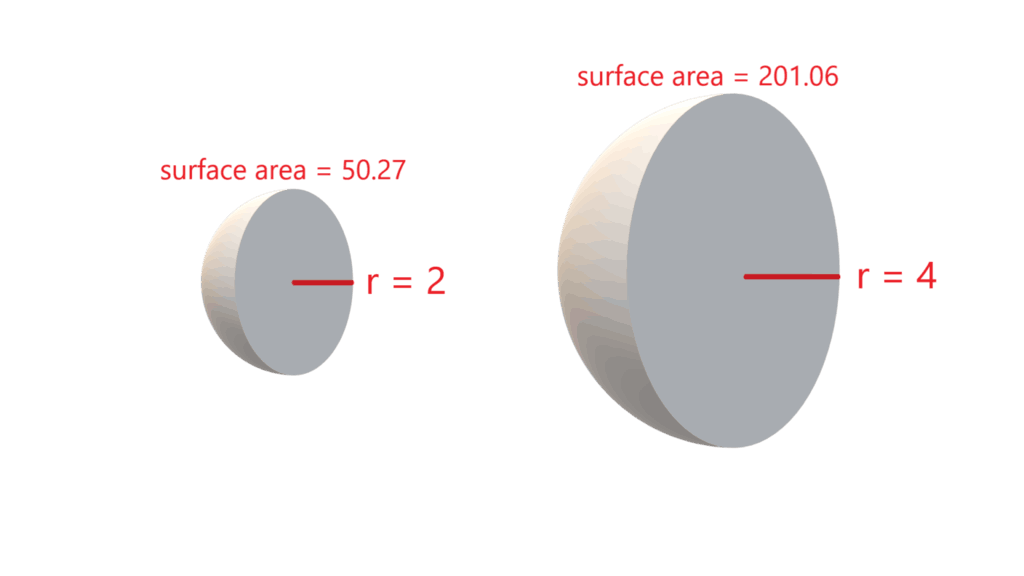
Questions?
- is it that as there are overlapping harmonics in common between the two notes, the more consonant they sound together?
Resources
- Acoustic room treatment software
- need 2 special kind of microphones explicitly for this purpose, which cost around $200 total
Related
vibrato
Children
Backlinks