Fractals
Fractals are defined as "a rough or fragmented geometric shape that can be split into parts, each of which is (at least approximately) a reduced-size copy of the whole."
Fractals are phenomenal, because what produces such a complex pattern arises from such a simple mathematical function
- "think not of what you see, but what it took to produce what you see"
Roughness is a key factor of fractals. Consider that if we had a perfectly straight coastline, there would be no apparent fractal.
Prices of a commodity (like cotton) takes a fractal pattern; the graph of one day prices looks like a graph of one year, which looks like a graph of 10 years
In nature, a fractal can be thought of an a solution that natural selection has come up with over and over again.
Other examples:
- Trees - You will find fractals at every level of the forest ecosystem from seeds and pinecones, to branches and leaves
- River Deltas
- Clouds
- Mountains
- Flowers
- Coastal areas
- Spider web
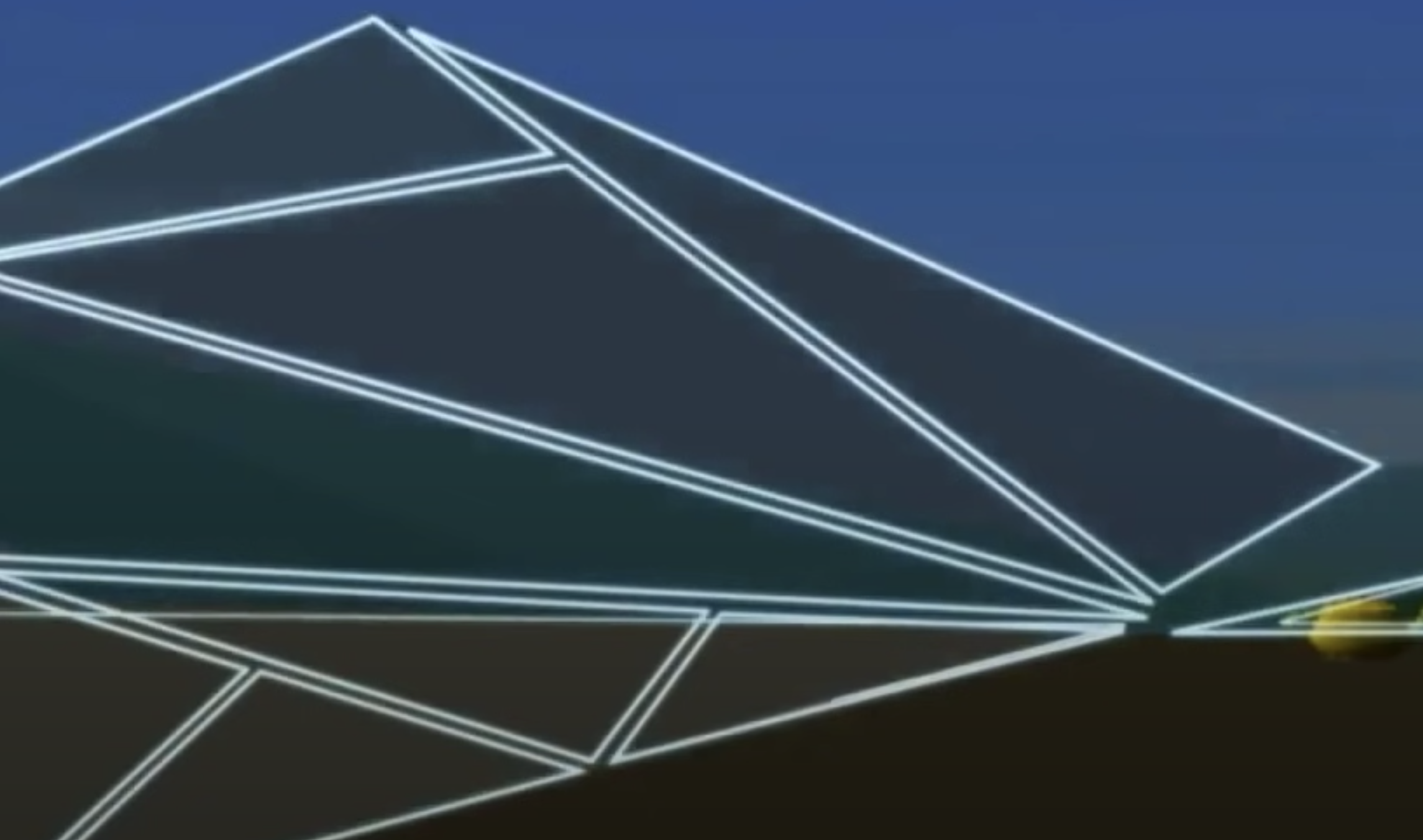
You can create a fractal by taking a smooth-looking shape and breaking it into pieces over and over again.
- ex. To make a mountain range, all you'd do is start with a few very broad triangles. Within each triangle, break it apart into smaller triangles, and continue doing this again and again until the shape starts to formulate.
UE Resources
Backlinks